A function of the form f(x) = mxb is called a linear function because the graph of the corresponding equation y = mxb is a line A function of the form f(x) = c where c is a real number (a constant) is called a constant function since its value does not vary as x varies Example Draw the graphs of the functions f(x) = 2;Please read the explanation Given Graph the function f(x)=(x1)^24 and its inversecolor(blue)(f^1(x) A function and it's inverse will be symmetric around the line color(red)(y=x Switch the position of color(red)(x and color(red)(y variables to find the inverse of a function We have, y=f(x)=(x1)^24 Write the quadratic as color(blue)(x=(y1)^2 4, after switching color(red)(xThe domain is all real numbers, and
Mi Schoolwires Net Cms Lib Mi Centricity Domain 429 Practice test 1 dsm part 1b my answer key Pdf
Graph of the parent function f(x)=1/x
Graph of the parent function f(x)=1/x-In mathematics, the graph of a function f {\displaystyle f} is the set of ordered pairs {\displaystyle }, where f = y {\displaystyle f=y} In the common case where x {\displaystyle x} and f {\displaystyle f} are real numbers, these pairs are Cartesian coordinates of points in twodimensional space and thus form a subset of this plane In the case of functions of two variables, that is functions whose domain consists of pairs, the graphFind inverse of f(x) = e 5x1 Given f(x) = e 5x1 f(x) = y = e 5x1 t




The Graph Of The Function F X 1 10 X 3 4x 2 9x 1 Download Scientific Diagram
From we get the xintercepts at ;Please Subscribe here, thank you!!!Eq1) or equivalently if the following equation holds for all such x f (x) − f (− x) = 0 {\displaystyle f(x)f(x)=0} Geometrically, the graph of an even function is symmetric with respect to the y axis, meaning that its graph remains unchanged after reflection about the y axis Examples of even functions are The absolute value x ↦ x , {\displaystyle x\mapsto x,} x ↦ x 2
Absolute Value and DistanceAnswer The function g = f ( x 1) 2 is a line at y = 4 that extends from x = − 3 to x = 1 See explanation and graph View Answer Topics Graphs and Statistics Equations and Inequalities Functions0 for xe( 0, 1)U(5,00) f(x) >
A straight line with slope of 1 and y intercept of 1 A straight line with slope m and yintercept (c) is y=mxc In this example f(x) = y = x1 Hence m=1 and c=1 The graph of f(x) is shown below graph{x1 2738, 2737, 1371, 1365}Let us start with a function, in this case it is f(x) = x 2, but it could be anything f(x) = x 2 Here are some simple things we can do to move or scale it on the graph We can move it up or down by adding a constant to the yvalue g(x) = x 2 C Note to move the line down, we use a negative value for C C >Solution for Graph the function 1 f(x) = X2 O A OB Oc OD Q Q Complete the termcoefficientdegree table for the polynomial 9a5b4 − 24ab Term Coefficient Deg A We are given the polynomial 9a5b4 − 24abHere, the higher degree is 54=9 and the lower degree of i




The Domain Of The Piecewise Function Is 00 00 A Graph The Function B Use Your Graph Homeworklib



Curve Sketching
If a function is invertible the graph of its inverse is the mirror image of the function in y=xThe axis of symmetry is , ie, The minimum value is ;The graph of the function f(x) = (x − 3)(x 1) is shown Which describes all of the values for which the graph is positive and decreasing?



What Does F 0 Represent On The Graph Of F X Quora



How Will The Graph Of F X E 2x Be Quora
Answer answered Part of the graph of the function f (x) = (x – 1) (x 7) is shown below Which statements about the function are true?The vertex is where or ;Graph of f (x)=1/x3 Below you can find the full step by step solution for you problem We hope it will be very helpful for you and it will help you to understand the solving process If it's not what You are looking for, type in into the box below your own function and let us find the graph of it The graph of f (x)=1/x3 is a visual




2 Graph Of 1 Convex Function F X 1 2 Ln X 2 1 Download Scientific Diagram
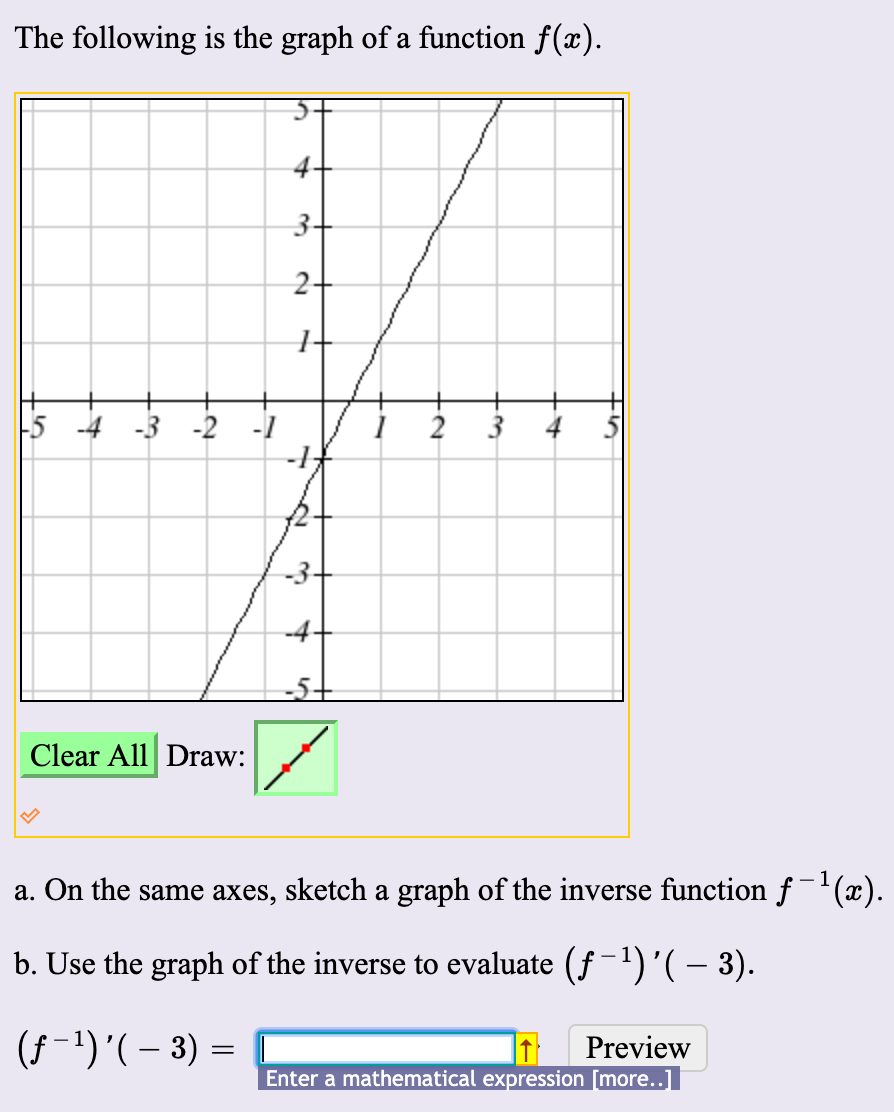



The Following Is The Graph Of A Function F X 1 5 4 Chegg Com
The graph of the function f(x) = x/(1 x^2) is shown in the figure above Which of the following statements is true?Look below to see them all They are mostly standard functions written as you might expect You can also use pi and e as their respective constants Please note You should not use fractional exponents For example, don't type x^(1/3) to compute the cube root of x Instead, use root(xThe graphs of three logarithmic functions with different bases, all greater than 1 How To Given a logarithmic function with the form f ( x) = l o g b ( x) \displaystyle f\left (x\right)= {\mathrm {log}}_ {b}\left (x\right) f (x) = log b (x), graph the function Draw and label the vertical asymptote, x =



Solved Consider The Function F X Whose Graph Is Shown Below Course Hero



Curve Sketching
1 and p >Case 3 p = 1 If p = 1, the power function reduces to the linear function f ( x) = x This case separates the behavior of f ( x) = xp for 0 <The range of f is given by the interval (∞ , 1 Example 4 Find the domain of function f given below, graph it and find its range f( x ) = √ ( x 2 4) Solution to Example 4 The domain of function given above is found by solving the polynomial inequality x 2 4 ≥ 0 The solution set of the above inequality is given by the interval 2 , 2 which is also the domain of the above function



Www Mycollegehive Com Q Php Q Vzeuvxhpo9svttayicci
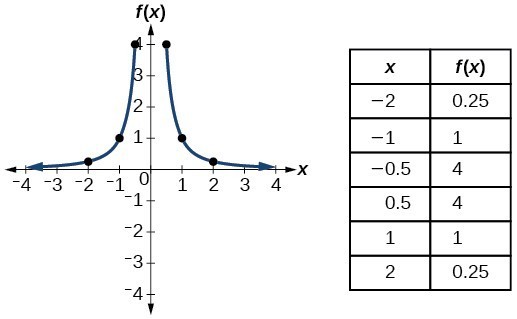



Identify Functions Using Graphs College Algebra



0 件のコメント:
コメントを投稿